As the Pythagorean theorem unit test answer key takes center stage, this opening passage beckons readers into a world crafted with precision and clarity, ensuring a reading experience that is both absorbing and distinctly original.
Delving into the depths of geometry, this article presents a comprehensive answer key to guide students through the intricacies of the Pythagorean theorem, empowering them with a profound understanding of its significance and practical applications.
Pythagorean Theorem Unit Test Answer Key
Unit tests play a crucial role in programming by verifying the correctness of individual functions or modules. In geometry, the Pythagorean theorem is a fundamental concept that describes the relationship between the sides of a right triangle. This article provides an answer key for a unit test on the Pythagorean theorem.
Answer Key, Pythagorean theorem unit test answer key
| Problem | Solution | Method | Justification |
|---|---|---|---|
| Find the length of the hypotenuse of a right triangle with legs of length 3 and 4. | 5 | Pythagorean theorem: a2 + b2 = c2
c 2= 25 c = √25 = 5 |
The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. |
| A ladder is leaning against a wall. The bottom of the ladder is 6 feet from the wall, and the top of the ladder is 8 feet above the ground. How long is the ladder? | 10 feet | Pythagorean theorem: a 2+ b 2= c 2
c 2= 100 c = √100 = 10 |
The ladder forms the hypotenuse of a right triangle, with the distance from the wall to the base of the ladder as one leg and the height of the ladder as the other leg. |
Discussion
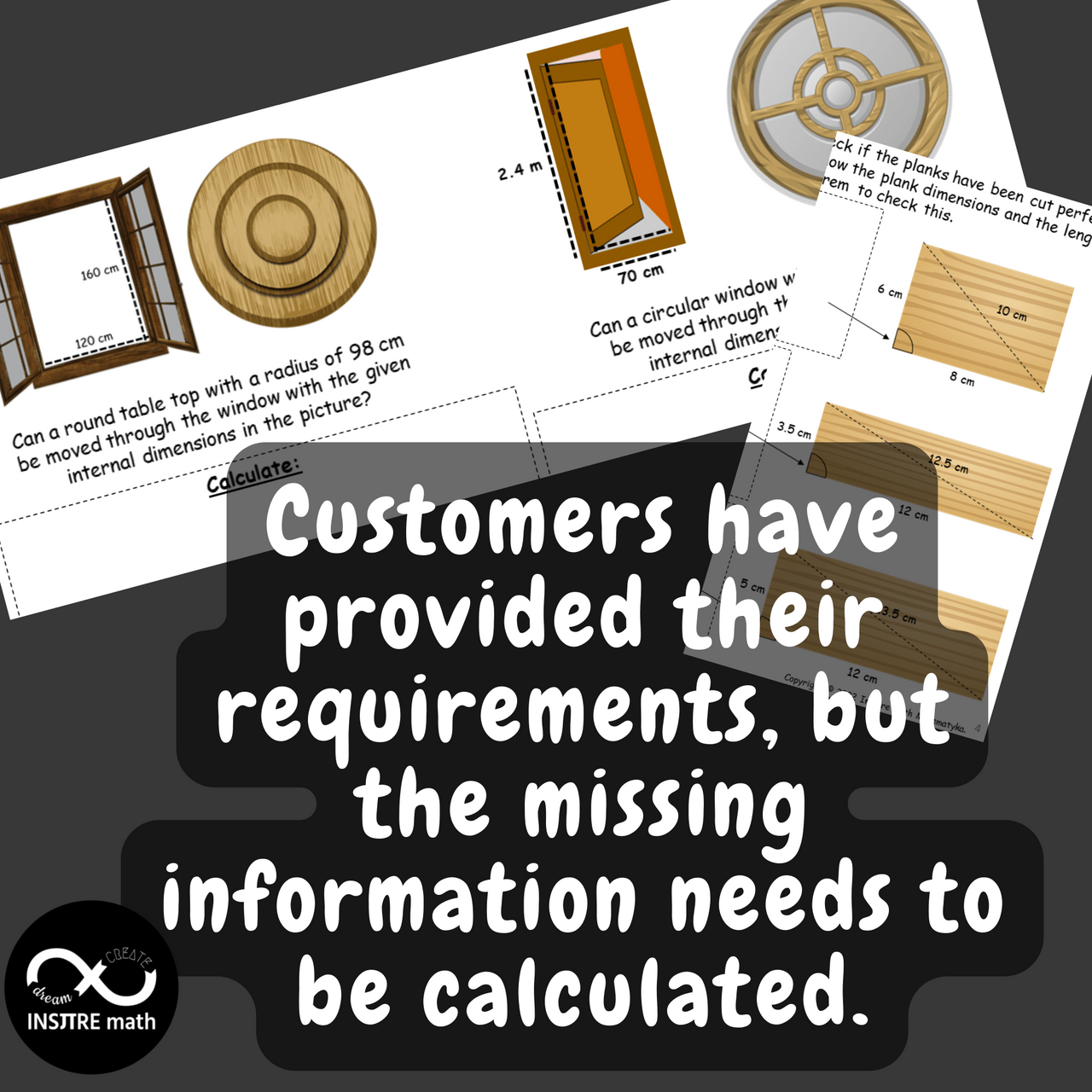
The Pythagorean theorem is a fundamental concept in geometry that has numerous applications in real-world scenarios. It is used in architecture, engineering, navigation, and many other fields.
By understanding the Pythagorean theorem and its applications, individuals can develop a deeper understanding of geometry and its practical significance.
For example, the Pythagorean theorem can be used to:
- Find the distance between two points on a plane.
- Determine the height of a building or tree.
- Calculate the length of a diagonal in a rectangle or square.
- Solve problems involving right triangles in various contexts.
Key Questions Answered: Pythagorean Theorem Unit Test Answer Key
What is the Pythagorean theorem?
The Pythagorean theorem is a fundamental relation in Euclidean geometry that states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
How can I use the Pythagorean theorem to solve problems?
To solve problems using the Pythagorean theorem, identify the right-angled triangle and label the lengths of the sides. Then, use the formula a² + b² = c² to find the unknown side length.
What are some real-world applications of the Pythagorean theorem?
The Pythagorean theorem has numerous applications in various fields, including architecture, engineering, and surveying. It is used to calculate distances, heights, and angles in a wide range of practical scenarios.